The Quantum

by John Gribbin from SkyBooksUSA Website Contents Quantum Early History Planck’s Contribution Einstein Contribution Bohr Atom Wave Mechanics What is Matter Matrix Mechanics Quantum Meaning Uncertainties Bose-Einstein Quantum results Developments Electrons The Future Quantum Computers Quantum Time Waits for No Cosmos Return to Quantum Return to Time Travel Return to Temas / Paraciencia Quantum Quantum Theory, also quantum mechanics, in physics, a theory based on using the concept of the quantum unit to describe the dynamic properties of subatomic particles and the interactions of matter and radiation. The foundation was laid by the German physicist Max Planck, who postulated in 1900 that energy can be emitted or absorbed by matter only in small, discrete units called quanta. Also fundamental to the development of quantum mechanics was the uncertainty principle, formulated by the German physicist Werner Heisenberg in 1927, which states that the position and momentum of a subatomic particle cannot be specified simultaneously. 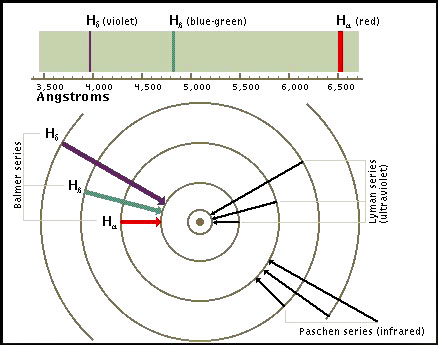Spectral Lines of Atomic Hydrogen: When an electron makes a transition from one energy level to another, the electron emits a photon with a particular energy. These photons are then observed as emission lines using a spectroscope. The Lyman series involves transitions to the lowest or ground state energy level. Transitions to the second energy level are called the Balmer series. These transitions involve frequencies in the visible part of the spectrum. In this frequency range each transition is characterized by a different color. Go Back Early History In the 18th and 19th centuries, Newtonian, or classical, mechanics appeared to provide a wholly accurate description of the motions of bodies—for example, planetary motion. In the late 19th and early 20th centuries, however, experimental findings raised doubts about the completeness of Newtonian theory. Among the newer observations were the lines that appear in the spectra of light emitted by heated gases, or gases in which electric discharges take place. From the model of the atom developed in the early 20th century by the English physicist Ernest Rutherford, in which negatively charged electrons circle a positive nucleus in orbits prescribed by Newton’s laws of motion, scientists had also expected that the electrons would emit light over a broad frequency range, rather than in the narrow frequency ranges that form the lines in a spectrum. Another puzzle for physicists was the coexistence of two theories of light: the corpuscular theory, which explains light as a stream of particles, and the wave theory, which views light as electromagnetic waves. A third problem was the absence of a molecular basis for thermodynamics. In his book Elementary Principles in Statistical Mechanics (1902), the American mathematical physicist J. Willard Gibbs conceded the impossibility of framing a theory of molecular action that reconciled thermodynamics, radiation, and electrical phenomena as they were then understood. Go Back Planck’s Contribution At the turn of the century, physicists did not yet clearly recognize that these and other difficulties in physics were in any way related. The first development that led to the solution of these difficulties was Planck’s introduction of the concept of the quantum, as a result of physicists’ studies of blackbody radiation during the closing years of the 19th century. (The term blackbody refers to an ideal body or surface that absorbs all radiant energy without any reflection.) A body at a moderately high temperature — a "red heat" — gives off most of its radiation in the low frequency (red and infrared) regions; a body at a higher temperature — "white heat" — gives off comparatively more radiation in higher frequencies (yellow, green, or blue). During the 1890s physicists conducted detailed quantitative studies of these phenomena and expressed their results in a series of curves or graphs. The classical, or prequantum, theory predicted an altogether different set of curves from those actually observed. What Planck did was to devise a mathematical formula that described the curves exactly; he then deduced a physical hypothesis that could explain the formula. His hypothesis was that energy is radiated only in quanta of energy hu, where u is the frequency and h is the quantum action, now known as Planck’s constant. Go Back Einstein Contribution The next important developments in quantum mechanics were the work of German-born American physicist and Nobel laureate Albert Einstein. He used Planck’s concept of the quantum to explain certain properties of the photoelectric effect—an experimentally observed phenomenon in which electrons are emitted from metal surfaces when radiation falls on these surfaces. According to classical theory, the energy, as measured by the voltage of the emitted electrons, should be proportional to the intensity of the radiation. The energy of the electrons, however, was found to be independent of the intensity of radiation—which determined only the number of electrons emitted—and to depend solely on the frequency of the radiation. The higher the frequency of the incident radiation, the greater is the electron energy; below a certain critical frequency no electrons are emitted. These facts were explained by Einstein by assuming that a single quantum of radiant energy ejects a single electron from the metal. The energy of the quantum is proportional to the frequency, and so the energy of the electron depends on the frequency. Go Back Bohr Atom In 1911 Rutherford established the existence of the atomic nucleus. He assumed, on the basis of experimental evidence obtained from the scattering of alpha particles by the nuclei of gold atoms, that every atom consists of a dense, positively charged nucleus, surrounded by negatively charged electrons revolving around the nucleus as planets revolve around the sun. The classical electromagnetic theory developed by the British physicist James Clerk Maxwell unequivocally predicted that an electron revolving around a nucleus will continuously radiate electromagnetic energy until it has lost all its energy, and eventually will fall into the nucleus. Thus, according to classical theory, an atom, as described by Rutherford, is unstable. This difficulty led the Danish physicist Niels Bohr, in 1913, to postulate that in an atom the classical theory does not hold, and that electrons move in fixed orbits. Every change in orbit by the electron corresponds to the absorption or emission of a quantum of radiation. The application of Bohr’s theory to atoms with more than one electron proved difficult. The mathematical equations for the next simplest atom, the helium atom, were solved during the 1910s and 1920s, but the results were not entirely in accordance with experiment. For more complex atoms, only approximate solutions of the equations are possible, and these are only partly concordant with observations. Go Back Wave Mechanics The French physicist Louis Victor de Broglie suggested in 1924 that because electromagnetic waves show particle characteristics, particles should, in some cases, also exhibit wave properties. This prediction was verified experimentally within a few years by the American physicists Clinton Joseph Davisson and Lester Halbert Germer and the British physicist George Paget Thomson. They showed that a beam of electrons scattered by a crystal produces a diffraction pattern characteristic of a wave (see Diffraction). The wave concept of a particle led the Austrian physicist Erwin Schrödinger to develop a so-called wave equation to describe the wave properties of a particle and, more specifically, the wave behavior of the electron in the hydrogen atom. Although this differential equation was continuous and gave solutions for all points in space, the permissible solutions of the equation were restricted by certain conditions expressed by mathematical equations called eigenfunctions (German eigen, "own"). The Schrödinger wave equation thus had only certain discrete solutions; these solutions were mathematical expressions in which quantum numbers appeared as parameters. (Quantum numbers are integers developed in particle physics to give the magnitudes of certain characteristic quantities of particles or systems.) The Schrödinger equation was solved for the hydrogen atom and gave conclusions in substantial agreement with earlier quantum theory. Moreover, it was solvable for the helium atom, which earlier theory had failed to explain adequately, and here also it was in agreement with experimental evidence. The solutions of the Schrödinger equation also indicated that no two electrons could have the same four quantum numbers—that is, be in the same energy state. This rule, which had already been established empirically by Austro-American physicist and Nobel laureate Wolfgang Pauli in 1925, is called the exclusion principle. Go Back What is Matter In the 20th century, physicists discovered that matter behaved as both a wave and a particle. Austrian physicist and Nobel Prize winner Erwin Schrödinger discussed this apparent paradox in a lecture in Geneva, Switzerland, in 1952. A condensed and translated version of his lecture appeared in Scientific American the following year. What Is Matter? The wave-particle dualism afflicting modern physics is best resolved in favor of waves, believes the author, but there is no clear picture of matter on which physicists can agree Fifty years ago science seemed on the road to a clear-cut answer to the ancient question which is the title of this article. It looked as if matter would be reduced at last to its ultimate building blocks—to certain submicroscopic but nevertheless tangible and measurable particles. But it proved to be less simple than that. Today a physicist no longer can distinguish significantly between matter and something else. We no longer contrast matter with forces or fields of force as different entities; we know now that these concepts must be merged. It is true that we speak of "empty" space (i.e., space free of matter), but space is never really empty, because even in the remotest voids of the universe there is always starlight—and that is matter. Besides, space is filled with gravitational fields, and according to Einstein gravity and inertia cannot very well be separated. Thus the subject of this article is in fact the total picture of space-time reality as envisaged by physics. We have to admit that our conception of material reality today is more wavering and uncertain than it has been for a long time. We know a great many interesting details, learn new ones every week. But to construct a clear, easily comprehensible picture on which all physicists would agree—that is simply impossible. Physics stands at a grave crisis of ideas. In the face of this crisis, many maintain that no objective picture of reality is possible. However, the optimists among us (of whom I consider myself one) look upon this view as a philosophical extravagance born of despair. We hope that the present fluctuations of thinking are only indications of an upheaval of old beliefs which in the end will lead to something better than the mess of formulas which today surrounds our subject. Since the picture of matter that I am supposed to draw does not yet exist, since only fragments of it are visible, some parts of this narrative may be inconsistent with others. Like Cervantes’ tale of Sancho Panza, who loses his donkey in one chapter but a few chapters later, thanks to the forgetfulness of the author, is riding the dear little animal again, our story has contradictions. We must start with the well-established concept that matter is composed of corpuscles or atoms, whose existence has been quite "tangibly" demonstrated by many beautiful experiments, and with Max Planck’s discovery that energy also comes in indivisible units, called quanta, which are supposed to be transferred abruptly from one carrier to another. But then Sancho Panza’s donkey will return. For I shall have to ask you to believe neither in corpuscles as permanent individuals nor in the suddenness of the transfer of an energy quantum. Discreteness is present, but not in the traditional sense of discrete single particles, let alone in the sense of abrupt processes. Discreteness arises merely as a structure from the laws governing the phenomena. These laws are by no means fully understood; a probably correct analogue from the physics of palpable bodies is the way various partial tones of a bell derive from its shape and from the laws of elasticity to which, of themselves, nothing discontinuous adheres. The idea that matter is made up of ultimate particles was advanced as early as the fifth century B.C. by Leucippus and Democritus, who called these particles atoms. The corpuscular theory of matter was lifted to physical reality in the theory of gases developed during the 19th century by James Clerk Maxwell and Ludwig Boltzmann. The concept of atoms and molecules in violent motion, colliding and rebounding again and again, led to full comprehension of all the properties of gases: their elastic and thermal properties, their viscosity, heat conductivity and diffusion. At the same time it led to a firm foundation of the mechanical theory of heat, namely, that heat is the motion of these ultimate particles, which becomes increasingly violent with rising temperature. Within one tremendously fertile decade at the turn of the century came the discoveries of X-rays, of electrons, of the emission of streams of particles and other forms of energy from the atomic nucleus by radioactive decay, of the electric charges on the various particles. The masses of these particles, and of the atoms themselves, were later measured very precisely, and from this was discovered the mass defect of the atomic nucleus as a whole. The mass of a nucleus is less than the sum of the masses of its component particles; the lost mass becomes the binding energy holding the nucleus firmly together. This is called the packing effect. The nuclear forces of course are not electrical forces—those are repellent—but are much stronger and act only within very short distances, about 10-13 centimeter. Here I am already caught in a contradiction. Didn’t I say at the beginning that we no longer assume the existence of force fields apart from matter? I could easily talk myself out of it by saying: Well, the force field of a particle is simply considered a part of it. But that is not the fact. The established view today is rather that everything is at the same time both particle and field. Everything has the continuous structure with which we are familiar in fields, as well as the discrete structure with which we are equally familiar in particles. This concept is supported by innumerable experimental facts and is accepted in general, though opinions differ on details, as we shall see. In the particular case of the field of nuclear forces, the particle structure is more or less known. Most likely the continuous force field is represented by the so-called pi mesons. On the other hand, the protons and neutrons, which we think of as discrete particles, indisputably also have a continuous wave structure, as is shown by the interference patterns they form when diffracted by a crystal. The difficulty of combining these two so very different character traits in one mental picture is the main stumbling-block that causes our conception of matter to be so uncertain. Neither the particle concept nor the wave concept is hypothetical. The tracks in a photographic emulsion or in a Wilson cloud chamber leave no doubt of the behavior of particles as discrete units. The artificial production of nuclear particles is being attempted right now with terrific expenditure, defrayed in the main by the various state ministries of defense. It is true that one cannot kill anybody with one such racing particle, or else we should all be dead by now. But their study promises, indirectly, a hastened realization of the plan for the annihilation of mankind which is so close to all our hearts. You can easily observe particles yourself by looking at a luminous numeral of your wrist watch in the dark with a magnifying glass. The luminosity surges and undulates, just as a lake sometimes twinkles in the sun. The light consists of sparklets, each produced by a so-called alpha particle (helium nucleus) expelled by a radioactive atom which in this process is transformed into a different atom. A specific device for detecting and recording single particles is the Geiger-Müller counter. In this short résumé I cannot possibly exhaust the many ways in which we can observe single particles. Now to the continuous field or wave character of matter. Wave structure is studied mainly by means of diffraction and interference—phenomena which occur when wave trains cross each other. For the analysis and measurement of light waves the principal device is the ruled grating, which consists of a great many fine, parallel, equidistant lines, closely engraved on a specular metallic surface. Light impinging from one direction is scattered by them and collected in different directions depending on its wavelength. But even the finest ruled gratings we can produce are too coarse to scatter the very much shorter waves associated with matter. The fine lattices of crystals, however, which Max von Laue first used as gratings to analyze the very short X-rays, will do the same for "matter waves." Directed at the surface of a crystal, high-velocity streams of particles manifest their wave nature. With crystal gratings physicists have diffracted and measured the wavelengths of electrons, neutrons and protons. What does Planck’s quantum theory have to do with all this? Planck told us in 1900 that he could comprehend the radiation from red-hot iron, or from an incandescent star such as the sun, only if this radiation was produced in discrete portions and transferred in such discrete quantities from one carrier to another (e.g., from atom to atom). This was extremely startling, because up to that time energy had been a highly abstract concept. Five years later Einstein told us that energy has mass and mass is energy; in other words, that they are one and the same. Now the scales begin to fall from our eyes: our dear old atoms, corpuscles, particles are Planck’s energy quanta. The carriers of those quanta are themselves quanta. One gets dizzy. Something quite fundamental must lie at the bottom of this, but it is not surprising that the secret is not yet understood. After all, the scales did not fall suddenly. It took 20 or 30 years. And perhaps they still have not fallen completely. The next step was not quite so far reaching, but important enough. By an ingenious and appropriate generalization of Planck’s hypothesis Niels Bohr taught us to understand the line spectra of atoms and molecules and how atoms were composed of heavy, positively charged nuclei with light, negatively charged electrons revolving around them. Each small system—atom or molecule—can harbor only definite discrete energy quantities, corresponding to its nature or its constitution. In transition from a higher to a lower "energy level" it emits the excess energy as a radiation quantum of definite wavelength, inversely proportional to the quantum given off. This means that a quantum of given magnitude manifests itself in a periodic process of definite frequency which is directly proportional to the quantum; the frequency equals the energy quantum divided by the famous Planck’s constant, h. According to Einstein a particle has the energy mc2, m being the mass of the particle and c the velocity of light. In 1925 Louis de Broglie drew the inference, which rather suggests itself, that a particle might have associated with it a wave process of frequency mc2 divided by h. The particle for which he postulated such a wave was the electron. Within two years the "electron waves" required by his theory were demonstrated by the famous electron diffraction experiment of C. J. Davisson and L. H. Germer. This was the starting point for the cognition that everything — anything at all — is simultaneously particle and wave field. Thus de Broglie’s dissertation initiated our uncertainty about the nature of matter. Both the particle picture and the wave picture have truth value, and we cannot give up either one or the other. But we do not know how to combine them. That the two pictures are connected is known in full generality with great precision and down to amazing details. But concerning the unification to a single, concrete, palpable picture opinions are so strongly divided that a great many deem it altogether impossible. I shall briefly sketch the connection. But do not expect that a uniform, concrete picture will emerge before you; and do not blame the lack of success either on my ineptness in exposition or your own denseness—nobody has yet succeeded. One distinguishes two things in a wave. First of all, a wave has a front, and a succession of wave fronts forms a system of surfaces like the layers of an onion. You are familiar with the two-dimensional analogue of the beautiful wave circles that form on the smooth surface of a pond when a stone is thrown in. The second characteristic of a wave, less intuitive, is the path along which it travels—a system of imagined lines perpendicular to the wave fronts. These lines are known as the wave "normals" or "rays." We can make the provisional assertion that these rays correspond to the trajectories of particles. Indeed, if you cut a small piece out of a wave, approximately 10 or 20 wavelengths along the direction of propagation and about as much across, such a "wave packet" would actually move along a ray with exactly the same velocity and change of velocity as we might expect from a particle of this particular kind at this particular place, taking into account any force fields acting on the particle. Here I falter. For what I must say now, though correct, almost contradicts this provisional assertion. Although the behavior of the wave packet gives us a more or less intuitive picture of a particle, which can be worked out in detail (e.g., the momentum of a particle increases as the wavelength decreases; the two are inversely proportional), yet for many reasons we cannot take this intuitive picture quite seriously. For one thing, it is, after all, somewhat vague, the more so the greater the wavelength. For another, quite often we are dealing not with a small packet but with an extended wave. For still another, we must also deal with the important special case of very small "packelets" which form a kind of "standing wave" which can have no wave fronts or wave normals. One interpretation of wave phenomena which is extensively supported by experiments is this: At each position of a uniformly propagating wave train there is a twofold structural connection of interactions, which may be distinguished as "longitudinal" and "transversal." The transversal structure is that of the wave fronts and manifests itself in diffraction and interference experiments; the longitudinal structure is that of the wave normals and manifests itself in the observation of single particles. However, these concepts of longitudinal and transversal structures are not sharply defined and absolute, since the concepts of wave front and wave normal are not, either. The interpretation breaks down completely in the special case of the standing waves mentioned above. Here the whole wave phenomenon is reduced to a small region of the dimensions of a single or very few wavelengths. You can produce standing water waves of a similar nature in a small basin if you dabble with your finger rather uniformly in its center, or else just give it a little push so that the water surface undulates. In this situation we are not dealing with uniform wave propagation; what catches the interest are the normal frequencies of these standing waves. The water waves in the basin are an analogue of a wave phenomenon associated with electrons, which occurs in a region just about the size of the atom. The normal frequencies of the wave group washing around the atomic nucleus are universally found to be exactly equal to Bohr’s atomic "energy levels" divided by Planck’s constant h. Thus the ingenious yet somewhat artificial assumptions of Bohr’s model of the atom, as well as of the older quantum theory in general, are superseded by the far more natural idea of de Broglie’s wave phenomenon. The wave phenomenon forms the "body" proper of the atom. It takes the place of the individual pointlike electrons which in Bohr’s model are supposed to swarm around the nucleus. Such pointlike single particles are completely out of the question within the atom, and if one still thinks of the nucleus itself in this way one does so quite consciously for reasons of expediency. What seems to me particularly important about the discovery that "energy levels" are virtually nothing but the frequencies of normal modes of vibration is that now one can do without the assumption of sudden transitions, or quantum jumps, since two or more normal modes may very well be excited simultaneously. The discreteness of the normal frequencies fully suffices—so I believe—to support the considerations from which Planck started and many similar and just as important ones—I mean, in short, to support all of quantum thermodynamics. The theory of quantum jumps is becoming more and more unacceptable, at least to me personally, as the years go on. Its abandonment has, however, far-reaching consequences. It means that one must give up entirely the idea of the exchange of energy in well-defined quanta and replace it with the concept of resonance between vibrational frequencies. Yet we have seen that because of the identity of mass and energy, we must consider the particles themselves as Planck’s energy quanta. This is at first frightening. For the substituted theory implies that we can no longer consider the individual particle as a well-defined permanent entity. That it is, in fact, no such thing can be reasoned in other ways. For one thing, there is Werner Heisenberg’s famous uncertainty principle, according to which a particle cannot simultaneously have a well-defined position and a sharply defined velocity. This uncertainty implies that we cannot be sure that the same particle could ever be observed twice. Another conclusive reason for not attributing identifiable sameness to individual particles is that we must obliterate their individualities whenever we consider two or more interacting particles of the same kind, e.g., the two electrons of a helium atom. Two situations which are distinguished only by the interchange of the two electrons must be counted as one and the same; if they are counted as two equal situations, nonsense obtains. This circumstance holds for any kind of particle in arbitrary numbers without exception. Most theoreticians will probably accept the foregoing reasoning and admit that the individual particle is not a well-defined permanent entity of detectable identity or sameness. Nevertheless this inadmissible concept of the individual particle continues to play a large role in their ideas and discussions. Even deeper rooted is the belief in "quantum jumps," which is now surrounded with a highly abstruse terminology whose common-sense meaning is often difficult to grasp. For instance, an important word in the standing vocabulary of quantum theory is "probability," referring to transition from one level to another. But, after all, one can speak of the probability of an event only assuming that, occasionally, it actually occurs. If it does occur, the transition must indeed be sudden, since intermediate stages are disclaimed. Moreover, if it takes time, it might conceivably be interrupted halfway by an unforeseen disturbance. This possibility leaves one completely at sea. The wave v. corpuscle dilemma is supposed to be resolved by asserting that the wave field merely serves for the computation of the probability of finding a particle of given properties at a given position if one looks for it there. But once one deprives the waves of reality and assigns them only a kind of informative role, it becomes very difficult to understand the phenomena of interference and diffraction on the basis of the combined action of discrete single particles. It certainly seems easier to explain particle tracks in terms of waves than to explain the wave phenomenon in terms of corpuscles. "Real existence" is, to be sure, an expression which has been virtually chased to death by many philosophical hounds. Its simple, naive meaning has almost become lost to us. Therefore I want to recall something else. I spoke of a corpuscle’s not being an individual. Properly speaking, one never observes the same particle a second time—very much as Heraclitus says of the river. You cannot mark an electron, you cannot paint it red. Indeed, you must not even think of it as marked; if you do, your "counting" will be false and you will get wrong results at every step—for the structure of line spectra, in thermodynamics and elsewhere. A wave, on the other hand, can easily be imprinted with an individual structure by which it can be recognized beyond doubt. Think of the beacon fires that guide ships at sea. The light shines according to a definite code; for example: three seconds light, five seconds dark, one second light, another pause of five seconds, and again light for three seconds—the skipper knows that is San Sebastian. Or you talk by wireless telephone with a friend across the Atlantic; as soon as he says, "Hello there, Edward Meier speaking," you know that his voice has imprinted on the radio wave a structure which can be distinguished from any other. But one does not have to go that far. If your wife calls, "Francis!" from the garden, it is exactly the same thing, except that the structure is printed on sound waves and the trip is shorter (though it takes somewhat longer than the journey of radio waves across the Atlantic). All our verbal communication is based on imprinted individual wave structures. And, according to the same principle, what a wealth of details is transmitted to us in rapid succession by the movie or the television picture! This characteristic, the individuality of the wave phenomenon, has already been found to a remarkable extent in the very much finer waves of particles. One example must suffice. A limited volume of gas, say helium, can be thought of either as a collection of many helium atoms or as a superposition of elementary wave trains of matter waves. Both views lead to the same theoretical results as to the behavior of the gas upon heating, compression, and so on. But when you attempt to apply certain somewhat involved enumerations to the gas, you must carry them out in different ways according to the mental picture with which you approach it. If you treat the gas as consisting of particles, then no individuality must be ascribed to them, as I said. If, however, you concentrate on the matter wave trains instead of on the particles, every one of the wave trains has a well-defined structure which is different from that of any other. It is true that there are many pairs of waves which are so similar to each other that they could change roles without any noticeable effect on the gas. But if you should count the very many similar states formed in this way as merely a single one, the result would be quite wrong. In spite of everything we cannot completely banish the concepts of quantum jump and individual corpuscle from the vocabulary of physics. We still require them to describe many details of the structure of matter. How can one ever determine the weight of a carbon nucleus and of a hydrogen nucleus, each to the precision of several decimals, and detect that the former is somewhat lighter than the 12 hydrogen nuclei combined in it, without accepting for the time being the view that these particles are something quite concrete and real? This view is so much more convenient than the roundabout consideration of wave trains that we cannot do without it, just as the chemist does not discard his valence-bond formulas, although he fully realizes that they represent a drastic simplification of a rather involved wave-mechanical situation. If you finally ask me: "Well, what are these corpuscles, really?" I ought to confess honestly that I am almost as little prepared to answer that as to tell where Sancho Panza’s second donkey came from. At the most, it may be permissible to say that one can think of particles as more or less temporary entities within the wave field whose form and general behavior are nevertheless so clearly and sharply determined by the laws of waves that many processes take place as if these temporary entities were substantial permanent beings. The mass and the charge of particles, defined with such precision, must then be counted among the structural elements determined by the wave laws. The conservation of charge and mass in the large must be considered as a statistical effect, based on the "law of large numbers." Go Back Matrix Mechanics Simultaneously with the development of wave mechanics, Heisenberg evolved a different mathematical analysis known as matrix mechanics. According to Heisenberg’s theory, which was developed in collaboration with the German physicists Max Born and Ernst Pascual Jordan, the formula was not a differential equation but a matrix: an array consisting of an infinite number of rows, each row consisting of an infinite number of quantities. Matrix mechanics introduced infinite matrices to represent the position and momentum of an electron inside an atom. Also, different matrices exist, one for each observable physical property associated with the motion of an electron, such as energy, position, momentum, and angular momentum. These matrices, like Schrödinger’s differential equations, could be solved; in other words, they could be manipulated to produce predictions as to the frequencies of the lines in the hydrogen spectrum and other observable quantities. Like wave mechanics, matrix mechanics was in agreement with the earlier quantum theory for processes in which the earlier quantum theory agreed with experiment; it was also useful in explaining phenomena that earlier quantum theory could not explain. Go Back Quantum Meaning Schrödinger subsequently succeeded in showing that wave mechanics and matrix mechanics are different mathematical versions of the same theory, now called quantum mechanics. Even for the simple hydrogen atom, which consists of two particles, both mathematical interpretations are extremely complex. The next simplest atom, helium, has three particles, and even in the relatively simple mathematics of classical dynamics, the three-body problem (that of describing the mutual interactions of three separate bodies) is not entirely soluble. The energy levels can be calculated accurately, however, even if not exactly. In applying quantum-mechanics mathematics to relatively complex situations, a physicist can use one of a number of mathematical formulations. The choice depends on the convenience of the formulation for obtaining suitable approximate solutions. Although quantum mechanics describes the atom purely in terms of mathematical interpretations of observed phenomena, a rough verbal description can be given of what the atom is now thought to be like. Surrounding the nucleus is a series of stationary waves; these waves have crests at certain points, each complete standing wave representing an orbit. The absolute square of the amplitude of the wave at any point is a measure of the probability that an electron will be found at that point at any given time. Thus, an electron can no longer be said to be at any precise point at any given time. Go Back Uncertainties The impossibility of pinpointing an electron at any precise time was analyzed by Heisenberg, who in 1927 formulated the uncertainty principle. This principle states the impossibility of simultaneously specifying the precise position and momentum of any particle. In other words, the more accurately a particle’s momentum is measured and known, the less accuracy there can be in the measurement and knowledge of its position. This principle is also fundamental to the understanding of quantum mechanics as it is generally accepted today: The wave and particle character of electromagnetic radiation can be understood as two complementary properties of radiation. Another way of expressing the uncertainty principle is that the wavelength of a quantum mechanical principle is inversely proportional to its momentum. As atoms are cooled they slow down and their corresponding wavelength grows larger. At a low enough temperature this wavelength is predicted to exceed the spacing between particles, causing atoms to overlap, becoming indistinguishable, and melding into a single quantum state. In 1995 a team of Colorado scientists, led by National Institutes of Standards and Technology physicist Eric Cornell and University of Colorado physicist Carl Weiman, cooled rubidium atoms to a temperature so low that the particles entered this merged state, known as a Bose-Einstein condensate. The condensate essentially behaves like one atom even though it is made up of thousands. Go Back Bose-Einstein - Physicists Condense Supercooled Atoms, Forming New State of Matter A team of Colorado physicists has cooled atoms of gas to a temperature so low that the particles entered a merged state, known as a "Bose-Einstein condensate." This phenomenon was first predicted about 70 years ago by the theories of German-born American physicist Albert Einstein and Indian physicist Satyendra Nath Bose. The condensed particles are considered a new state of matter, different from the common states of matter—gas, liquid, and solid—and from plasma, a high temperature, ionized form of matter that is found in the sun and other stars. Physicists have great expectations for the application of this discovery. Because the condensate essentially behaves like one atom even though it is made up of thousands, investigators should be able to measure interactions at the atomic and subatomic level that were previously extremely difficult, if not impossible, to study quantitatively. The condensate was detected June 5 by a Colorado team led by National Institutes of Standards and Technology physicist Eric Cornell and University of Colorado physicist Carl Wieman. Their discovery was reported in the journal Science on July 14. Cornell and Wieman formed their condensate from rubidium gas. Several groups of physicists, including the teams in Texas and Colorado and a group at the Massachusetts Institute of Technology, have been working to form pure condensate in recent years. The goal of the investigations has been to create a pure chunk of condensate out of atoms in an inert medium, such as a diffuse, nonreactive gas. The effort began when methods of cooling and trapping became refined enough that it seemed possible to reach the required conditions of temperature and density. The Colorado team used two techniques: first laser cooling and then evaporative cooling. The laser technique used laser light whose frequency was carefully tuned to interact with the rubidium atoms and gently reduce their speeds. A number of lasers were aimed at the gas to slow the motion of the atoms in different directions. The Colorado physicists then switched to evaporative cooling. In this method, the gas is "trapped" by a magnetic field that dwindles to zero at its center. Atoms that are moving wander out of the field, while the coldest atoms cluster at the center. Because a few very cold atoms could still escape at the zero field point of the trap, the physicists perfected their system by adding a second slowly circling magnetic field so that the zero point moved, not giving the atoms the chance to escape through it. Physicists will now begin to explore the properties of the condensate and see what other materials they can use to form it. One unusual characteristic of the condensate is that it is composed of atoms that have lost their individual identities. This is analogous to laser light, which is composed of light particles, or photons, that similarly have become indistinguishable and all behave in exactly the same manner. The laser has found a myriad of uses both in practical applications and in theoretical research, and the Bose-Einstein condensate may turn out to be just as important. Some scientists speculate that if a condensate can be readily produced and sustained, it could be used to miniaturize and speed up computer components to a scale and quickness not possible before. The prediction that a merged form of matter will emerge at extremely low temperatures is based on a number of aspects of the quantum theory. This theory governs the interaction of particles on a subatomic scale. The basic principle of quantum theory is that particles can only exist in certain discrete energy states. The exact "quantum state" of a particle takes into consideration such factors as the position of the particle and its "spin," which can only have certain discrete values. A particle’s spin categorizes it as either a boson or a fermion. Those two groups of particles behave according to different sets of statistical rules. Bosons have spins that are a constant number multiplied by an integer (e.g., 0, 1, 2, 3). Fermions have spins that are that same constant multiplied by an odd half-integer (1/2, 3/2, 5/2, etc.). Examples of fermions are the protons and neutrons that make up an atom’s nucleus, and electrons. Composite particles, such as nuclei and atoms, are classified as bosons or fermions based on the sum of the spins of their constituent particles. For instance, an isotope of helium called helium-4 turns out to be a bose particle. Helium-4 is made up of six fermi particles: two electrons orbiting a nucleus made up of two protons and two neutrons. Adding up six odd half-integers will yield a whole integer, making helium-4 a boson. The atoms of rubidium used in the Colorado experiment are bose particles as well. Only bose atoms may form a condensate, but they do so only at a sufficiently low temperature and high density. At their lab in Colorado, Cornell and Wieman cooled a rubidium gas down to a temperature as close to absolute zero, the temperature at which particles stop moving, as they could get. The slower the particles, the lower their momentum. In essence, the cooling brought the momentum of the gas particles closer and closer to precisely zero, as the temperature decreased to within a few billionths of a degree Kelvin. (Kelvin degrees are on the scale of degrees Celsius, but zero Kelvin is absolute zero, while zero Celsius is the freezing point of water.) As the temperature, and thus the momentum, of the gas particles dropped to an infinitesimal amount, the possible locations of the atom at any given moment increased proportionally. The goal of the experiment was to keep the gas atoms packed together closely enough that during this process—as their momentum got lower and lower, and their wavelengths got larger and larger—their waves would begin to overlap. This interplay of position and movement in three dimensions with the relative distances between particles is known as the phase-space density and is the key factor in forming a condensate. In essence, the momentum of the atoms would become so precisely pinpointed (near zero) that their position would become less and less certain and there would be a relatively large amount of space that would define each atom’s position. As the atoms slowed to almost a stop, their positions became so fuzzy that each atom came to occupy the same position as every other atom, losing their individual identity. This odd phenomenon is a Bose-Einstein condensate. As their experimental conditions neared the realm of Bose-Einstein condensation, Cornell and Wieman noticed an abrupt rise in the peak density of their sample, a type of discontinuity that strongly indicates a phase transition. The Colorado physicists estimated that after progressive evaporative cooling of the rubidium, they were left with a nugget of about 2,000 atoms of pure condensate. Cornell and Wieman then released the atoms from the "trap" in which they had been cooling and sent a pulse of laser light at the condensate, basically blowing it apart. They recorded an image of the expanding cloud of atoms. Prior to the light pulse, when the density dropped after the atoms were released, the physicists believed the temperature of the condensate fell to an amazing frigidity of 20 nanoKelvins (20 billionths of one degree above absolute zero). The image showed a larger, expanding sphere of particles with a smaller, more concentrated elliptical-looking center. Cornell and Wieman observed that when a gas is constrained and then released (in an extreme example, as in a bomb), thermodynamics specifies that it will expand outward equally in all directions regardless of the shape in which it had been contained. This occurs because the particles in that gas, even if the gas was very cold, were moving in all different directions with various energies when the gas was pushed outwards. This rule of uniform expansion does not hold for a Bose-Einstein condensate. Because the particles were all acting in exactly the same manner at the time of the light pulse, their expansion should give some indication of the shape of the space they had previously inhabited. The uneven, elliptical-looking clump of atoms in the center of the image recorded by Cornell and Wieman thus gave further proof that a condensate had formed. Bose-Einstein characteristics have been observed in other systems, specifically, in superfluid liquid helium-4 and in superconductors. It is believed that liquid helium-4 at a sufficiently low temperature is composed of two components mixed together, the colder of which is a Bose-Einstein condensate. Liquid helium-4, which at very low temperatures is also a superconductor of heat, behaves in dramatic ways, trickling up the sides of containers and rising in fountains. Electrical superconductors are also boson-related phenomena. In superconductors, which are also formed by supercooling, electrical resistance disappears. In this case it is the electrons within a substance’s atoms, rather than the atoms themselves, that condense. The electrons pair up, together forming a particle of zero spin. These paired electrons merge into an overall substance that flows freely through the superconductor, offering no resistance to electric current. Thus, once initiated, a current can flow indefinitely in a superconductor. Go Back Quantum results Quantum mechanics solved all of the great difficulties that troubled physicists in the early years of the 20th century. It gradually enhanced the understanding of the structure of matter, and it provided a theoretical basis for the understanding of atomic structure (see Atom and Atomic Theory) and the phenomenon of spectral lines: Each spectral line corresponds to the energy of a photon transmitted or absorbed when an electron makes a transition from one energy level to another. The understanding of chemical bonding was fundamentally transformed by quantum mechanics and came to be based on Schrödinger’s wave equations. New fields in physics emerged—condensed matter physics, superconductivity, nuclear physics, and elementary particle physics (see Physics)—that all found a consistent basis in quantum mechanics. Go Back Developments FURTHER DEVELOPMENTS: In the years since 1925, no fundamental deficiencies have been found in quantum mechanics, although the question of whether the theory should be accepted as complete has come under discussion. In the 1930s the application of quantum mechanics and special relativity to the theory of the electron (see Quantum Electrodynamics) allowed the British physicist Paul Dirac to formulate an equation that referred to the existence of the spin of the electron. It further led to the prediction of the existence of the positron, which was experimentally verified by the American physicist Carl David Anderson. The application of quantum mechanics to the subject of electromagnetic radiation led to explanations of many phenomena, such as bremsstrahlung (German, "braking radiation," the radiation emitted by electrons slowed down in matter) and pair production (the formation of a positron and an electron when electromagnetic energy interacts with matter). It also led to a grave problem, however, called the divergence difficulty: Certain parameters, such as the so-called bare mass and bare charge of electrons, appear to be infinite in Dirac’s equations. (The terms bare mass and bare charge refer to hypothetical electrons that do not interact with any matter or radiation; in reality, electrons interact with their own electric field.) This difficulty was partly resolved in 1947-49 in a program called renormalization, developed by the Japanese physicist Shin’ichirô Tomonaga, the American physicists Julian S. Schwinger and Richard Feynman, and the British physicist Freeman Dyson. In this program, the bare mass and charge of the electron are chosen to be infinite in such a way that other infinite physical quantities are canceled out in the equations. Renormalization greatly increased the accuracy with which the structure of atoms could be calculated from first principles. Go Back Electrons Theoretical physicist C. Llewellyn Smith discusses the discoveries that scientists have made to date about the electron and other elementary particles—subatomic particles that scientists believe cannot be split into smaller units of matter. Scientists have discovered what Smith refers to as sibling and cousin particles to the electron, but much about the nature of these particles is still a mystery. One way scientists learn about these particles is to accelerate them to high energies, smash them together, and then study what happens when they collide. By observing the behavior of these particles, scientists hope to learn more about the fundamental structures of the universe. Electrons: The First Hundred Years The discovery of the electron was announced by J. J. Thomson just over 100 years ago, on April 30, 1897. In the intervening years we have come to understand the mechanics that describe the behavior of electrons—and indeed of all matter on a small scale—which is called quantum mechanics. By exploiting this knowledge, we have learned to manipulate electrons and make devices of a tremendous practical and economic importance, such as transistors and lasers. Meanwhile, what have we learned of the nature of the electron itself? From the start, electrons were found to behave as elementary particles, and this is still the case today. We know that if the electron has any structure, it is on a scale of less than 10­18 m, i.e. less than 1 billionth of 1 billionth of a meter. However, a major complication has emerged. We have discovered that the electron has a sibling and cousins that are apparently equally fundamental. The sibling is an electrically neutral particle, called the neutrino, which is much lighter than the electron. The cousins are two electrically charged particles, called the mu and the tau, which also have neutral siblings. The mu and the tau seem to be identical copies of the electron, except that they are respectively 200 and 3,500 times heavier. Their role in the scheme of things and the origin of their different masses remain mysteries — just the sort of mysteries that particle physicists, who study the constituents of matter and the forces that control their behavior, wish to resolve. We therefore know of six seemingly fundamental particles, the electron, the mu, the tau and their neutral siblings, which—like the electron—do not feel the nuclear force, and incidentally are known generically as leptons. What about the constituents of atomic nuclei, which of course do feel the nuclear force? At first sight, nuclei are made of protons and neutrons, but these particles turned out not to be elementary. It was found that when protons and neutrons are smashed together, new particles are created. We now know that all these particles are made of more elementary entities, called quarks. In a collision, pairs of quarks and their antiparticles, called antiquarks, can be created: part of the energy (e) of the incoming particles is turned into mass (m) of these new particles, thanks to the famous equivalence e = mc2. The quarks in the projectiles and the created quark-antiquark pairs can then rearrange themselves to make various different sorts of new particles. Today, six types of quarks are known which, like the leptons (the electron and its relations) have simple properties, and could be elementary. In the past 30 years a recipe that describes the behavior of these particles has been developed. It is called the "Standard Model" of particle physics. However, we lack a real understanding of the nature of these particles, and the logic behind the Standard Model. What is wrong with the Standard Model? First, it does not consistently combine Einstein’s theory of the properties of space (called General Relativity) with a quantum mechanical description of the properties of matter. It is therefore logically incomplete. Second, it contains too many apparently arbitrary futures—it is too baroque, too byzantine—to be complete. It does not explain the role of the mu and the tau, or answer the question whether the fact that the numbers of leptons and quarks are the same—six each—is a coincidence, or an indication of a deep connection between these different types of particles. On paper, we can construct theories that give better answers and explanations, and in which there are such connections, but we do not know which, if any, of these theories is correct. Third, it has a missing, untested, element. This is not some minor detail, but a central element, namely a mechanism to generate the observed masses of the known particles, and hence also the different ranges of the known forces (long range for gravity and electromagnetism, as users of magnetic compasses know, but very short range for the nuclear and the so-called weak forces, although in every other respect these forces appear very similar). On paper, a possible mechanism is known, called the Higgs mechanism, after the British physicist Peter Higgs who invented it. But there are alternative mechanisms, and in any case the Higgs mechanism is a generic idea. We not only need to know if nature uses it, but if so, how it is realized in detail. Luckily the prospects of developing a deeper understanding are good. The way forward is to perform experiments that can distinguish the different possibilities. We know that the answer to the mystery of the origin of mass, and the different ranges of forces, and certain other very important questions, must lie in an energy range that will be explored in experiments at the Large Hadron Collider, a new accelerator now under construction at CERN [also known as the European Laboratory for Particle Physics] near Geneva. The fundamental tools on which experimental particle physics depends are large accelerators, like the Large Hadron Collider, which accelerate particles to very high energies and smash them together. By studying what happens in the collisions of these particles, which are typically electrons or protons (the nuclei of hydrogen atoms), we can learn about their natures. The conditions that are created in these collisions of particles existed just after the birth of the universe, when it was extremely hot and dense. Knowledge derived from experiments in particle physics is therefore essential input for those who wish to understand the structure of the universe as a whole, and how it evolved from an initial fireball into its present form. The Large Hadron Collider will therefore not only open up a large new window on the nature of matter, when it comes into operation in 2005, but also advance our understanding of the structure of the universe. However, although it will undoubtedly resolve some major questions and greatly improve our knowledge of nature, it would be very surprising if it established a "final theory." The only candidate theory currently known which appears to have the potential to resolve all the problems mentioned above—the reason for the existence of the mu and tau, reconciliation of Einstein’s general relativity with quantum mechanics, etc.—describes the electron and its relatives and the quarks, not as pointlike objects, but as different vibrating modes of tiny strings. However, these strings are so small (10-35 m) that they will never be observed directly. If this is so, the electron and the other known particles will continue forever to appear to be fundamental pointlike objects, even if the—currently very speculative—"string theory" scores enough successes to convince us that this is not the case! Go Back The Future FUTURE PROSPECTS: Quantum mechanics underlies current attempts to account for the strong nuclear force and to develop a unified theory for all the fundamental interactions of matter. Nevertheless, doubts exist about the completeness of quantum theory. The divergence difficulty, for example, is only partly resolved. Just as Newtonian mechanics was eventually amended by quantum mechanics and relativity, many scientists—and Einstein was among them—are convinced that quantum theory will also undergo profound changes in the future. Great theoretical difficulties exist, for example, between quantum mechanics and chaos theory, which began to develop rapidly in the 1980s. Ongoing efforts are being made by theorists such as the British physicist Stephen Hawking, to develop a system that encompasses both relativity and quantum mechanics. Go Back Quantum Computers Breakthroughs occurred in the area of quantum computing in the late 1990s. Quantum computers under development use components of a chloroform molecule (a combination of chlorine and hydrogen atoms) and a variation of a medical procedure called magnetic resonance imaging (MRI) to compute at a molecular level. Scientists used a branch of physics called quantum mechanics, which describes the activity of subatomic particles (particles that make up atoms), as the basis for quantum computing. Quantum computers may one day be thousands to millions of times faster than current computers, because they take advantage of the laws that govern the behavior of subatomic particles. These laws allow quantum computers to examine all possible answers to a query at one time. Future uses of quantum computers could include code breaking and large database queries. Go Back Quantum Time Waits for No Cosmos THE INTRIGUING notion that time might run backwards when the Universe collapses has run into difficulties. Raymond Laflamme, of the Los Alamos National Laboratory in New Mexico, has carried out a new calculation which suggests that the Universe cannot start out uniform, go through a cycle of expansion and collapse, and end up in a uniform state. It could start out disordered, expand, and then collapse back into disorder. But, since the COBE data show that our Universe was born in a smooth and uniform state, this symmetric possibility cannot be applied to the real Universe. Physicists have long puzzled over the fact that two distinct "arrows of time" both point in the same direction. In the everyday world, things wear out -- cups fall from tables and break, but broken cups never re- assemble themselves spontaneously. In the expanding Universe at large, the future is the direction of time in which galaxies are further apart. Many years ago, Thomas Gold suggested that these two arrows might be linked. That would mean that if and when the expansion of the Universe were to reverse, then the everyday arrow of time would also reverse, with broken cups re-assembling themselves. More recently, these ideas have been extended into quantum physics. There, the arrow of time is linked to the so-called "collapse of the wave function", which happens, for example, when an electron wave moving through a TV tube collapses into a point particle on the screen of the TV. Some researchers have tried to make the quantum description of reality symmetric in time, by including both the original state of the system (the TV tube before the electron passes through) and the final state (the TV tube after the electron has passed through) in one mathematical description. Murray Gell-Mann and James Hartle recently extended this idea to the whole Universe. They argued that if, as many cosmologists believe likely, the Universe was born in a Big Bang, will expand out for a finite time and then recollapse into a Big Crunch, the time-neutral quantum theory could describe time running backwards in the contracting half of its life. Unfortunately, Laflamme has now shown that this will not work. He has proved that if there are only small inhomogeneities present in the Big Bang, then they must get larger throughout the lifetime of the Universe, in both the expanding and the contracting phases. "A low entropy Universe at the Big Bang cannot come back to low entropy at the Big Crunch" (Classical and Quantum Gravity, vol 10 p L79). He has found time-asymmetric solutions to the equations -- but only if both Big Bang and Big Crunch are highly disordered, with the Universe more ordered in the middle of its life. Observations of the cosmic microwave background radiation show that the Universe emerged from the Big Bang in a very smooth and uniform state. This rules out the time-symmetric solutions. The implication is that even if the present expansion of the Universe does reverse, time will not run backwards and broken cups will not start re- assembling themselves.